Our real interest is in manifolds, and it is their classification that we will discuss in this section. In addition, we will refer to the concept of orientability here, which is defined for topological spaces in the next chapter.

As intuitive 2-dimensional guides, we have now utilized the sphere \({S^{2}}\), the torus \({T^{2}}\), and projective plane \({\mathbb{R}\textrm{P}^{2}}\). In fact, this essentially exhausts the topology of closed connected 2-manifolds, a full classification of which is:

• Orientable: the sphere, the torus, or the connected sum of tori
• Non-orientable: the projective plane, or a connected sum of projective planes

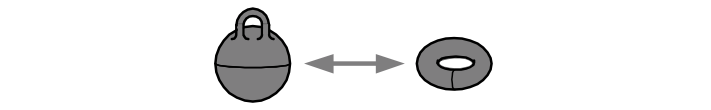
The Klein bottle, another well-known non-orientable surface (see the figure here), is homeomorphic to the connected sum \({\mathbb{R}\textrm{P}^{2}\#\mathbb{R}\textrm{P}^{2}}\). Any compact manifold with boundary is then obtained from these spaces by removing one or more open disks.

Another way of describing this classification scheme is using handles. A three-dimensional handlebody is a ball with \({g}\) handles attached to it, which is equivalent to the solid torus of genus \({g}\). Attaching a handle also refers to the corresponding operation on the boundary, i.e. adding a cylinder to a sphere by removing two disks. The concept of adding handles to a manifold is generalized in surgery theory.

The sphere with \({g}\) handles (equivalently, the torus with \({g}\) holes) is referred to as the surface of genus g, with the genus of a handlebody defined by that of its boundary. In this light, every closed connected 2-manifold is homeomorphic to a sphere, projective plane, or Klein bottle, possibly with some number of attached handles.

This simple classification scheme does not extend to higher dimensions, a clear indication of the limits of intuition in higher dimensional spaces. However, the following additional facts can be stated regarding the classification of manifolds:

• Every connected 1-manifold without boundary is homeomorphic to either the line \({\mathbb{R}}\) or the circle \({S^{1}}\)
• The classification of 3-manifolds was accomplished by the 2003 proof of Thurston’s geometrization conjecture
• It can be proved that the classification of manifolds of dimension four or greater is impossible
• Many properties can be proved for dimension five or greater, but not for four dimensions; for example, the simply-connected manifolds of dimension five or greater are completely classified

The recent classification of 3-manifolds is of particular interest, since it also proved the long-standing Poincaré conjecture, which generalized to arbitrary dimension states that every closed \({n}\)-manifold that is homotopy equivalent to the \({n}\)-sphere is homeomorphic to the \({n}\)-sphere. Since 1904 when the conjecture was first stated, it has been proven for every dimension except 3, with dimension 4 having been solved in 1982. Every compact orientable 3-manifold can be obtained by identifying the boundaries of two handlebodies of the same genus in some way; this is called a Heegaard splitting (AKA Heegaard decomposition). This does not amount to a classification, however, since different splittings can yield the same manifold.